Difference between revisions of "Delta hk"
From timescalewiki
| (6 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| − | Let $\mathbb{T}$ be a [[time scale]] and let $t,s \in \mathbb{T}$. The $h_k$ monomials are defined by the recurrence | + | Let $\mathbb{T}$ be a [[time scale]] and let $t,s \in \mathbb{T}$. The $h_k$ Taylor monomials are defined by the recurrence |
$$\left\{ \begin{array}{ll} | $$\left\{ \begin{array}{ll} | ||
| − | h_0(t,s)=1 \\ | + | h_0(t,s;\mathbb{T})=1 \\ |
| − | h_{ | + | h_{k+1}(t,s;\mathbb{T})= \displaystyle\int_s^t h_{k}(\tau,s;\mathbb{T}) \Delta \tau. |
\end{array} \right.$$ | \end{array} \right.$$ | ||
| Line 15: | Line 15: | ||
=Properties= | =Properties= | ||
| − | + | [[Relationship between delta hk and delta gk]]<br /> | |
=Examples= | =Examples= | ||
| Line 23: | Line 23: | ||
[[Delta gk]] | [[Delta gk]] | ||
| − | {{:Delta special functions footer}} | + | <center>{{:Delta special functions footer}}</center> |
| + | |||
| + | [[Category:specialfunction]] | ||
| + | [[Category:Definition]] | ||
Latest revision as of 14:13, 28 January 2023
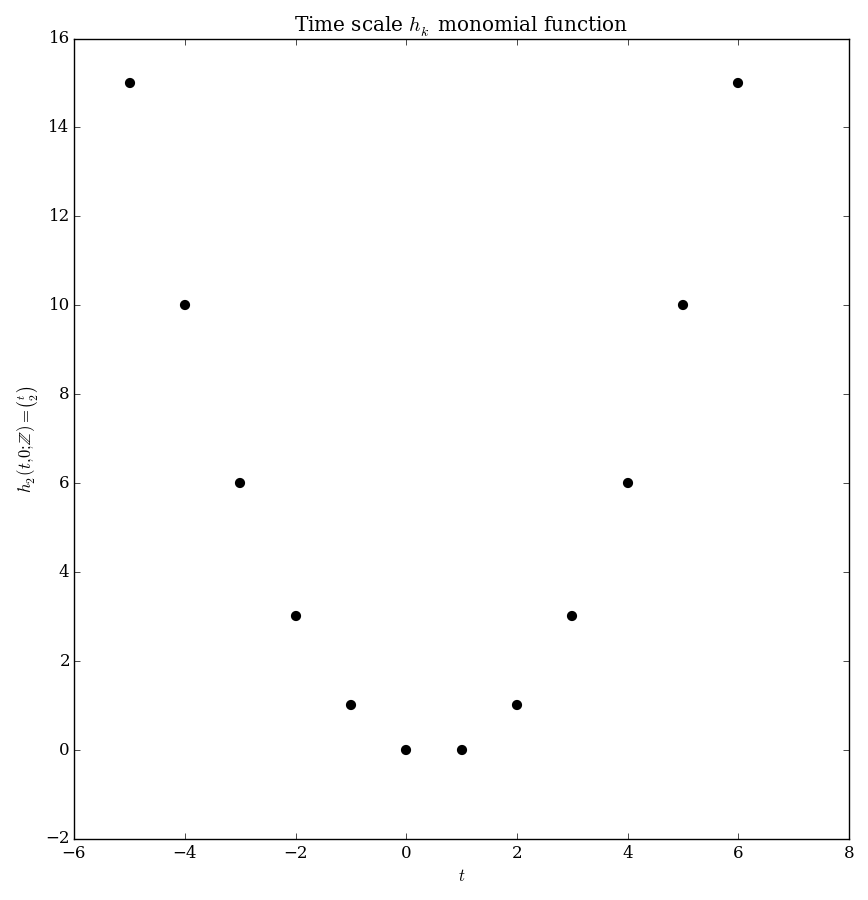
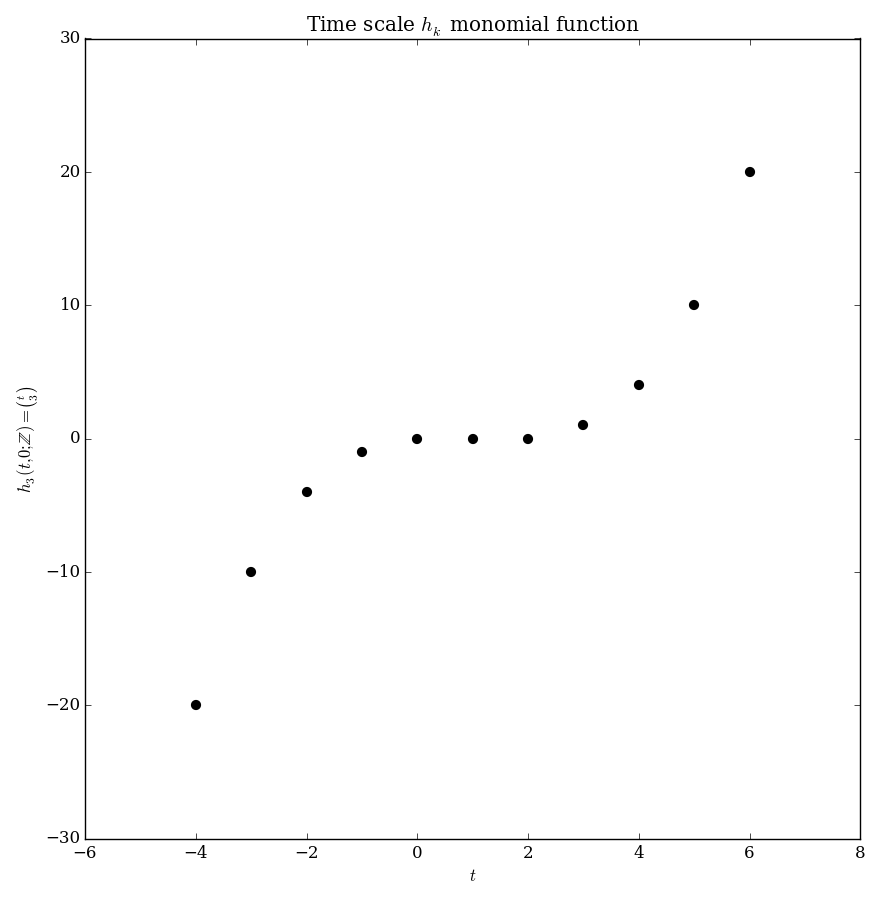
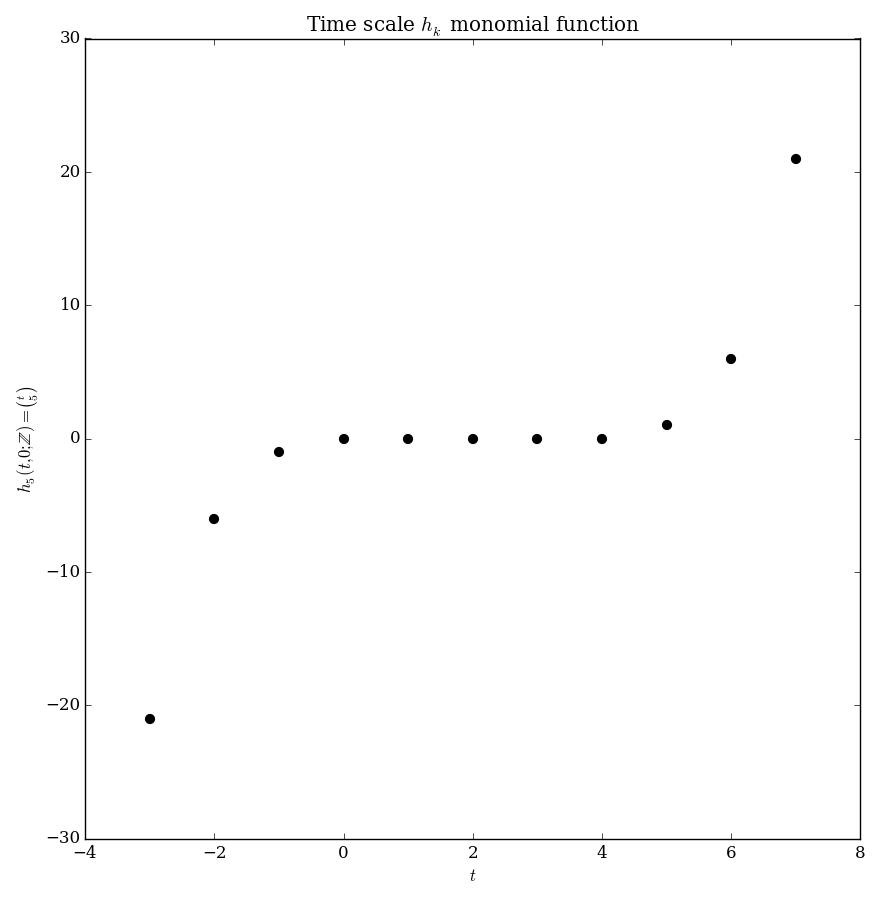
Let $\mathbb{T}$ be a time scale and let $t,s \in \mathbb{T}$. The $h_k$ Taylor monomials are defined by the recurrence $$\left\{ \begin{array}{ll} h_0(t,s;\mathbb{T})=1 \\ h_{k+1}(t,s;\mathbb{T})= \displaystyle\int_s^t h_{k}(\tau,s;\mathbb{T}) \Delta \tau. \end{array} \right.$$
Graph of $h_2(t,0;\mathbb{Z})$.
Graph of $h_3(t,0;\mathbb{Z})$.
Graph of $h_4(t,0;\mathbb{Z})$.
Graph of $h_5(t,0;\mathbb{Z})$.
Properties
Relationship between delta hk and delta gk
Examples
| $\mathbb{T}=$ | $h_k(t,s;\mathbb{T})=$ |
| $\mathbb{R}$ | $\dfrac{(t-s)^k}{k!}$ |
| $\mathbb{Z}$ | $\displaystyle{t-s \choose k} = \dfrac{(t-s)!}{k! (t-s-k)!}$ |
| $h\mathbb{Z}$ | $\dfrac{1}{k!} \displaystyle\prod_{\ell=0}^{k-1}(t-\ell h-s)$ |
| $\mathbb{Z}^2$ | |
| $\overline{q^{\mathbb{Z}}}, q > 1$ | $\displaystyle\prod_{n=0}^{k-1} \dfrac{t-q^ns}{\sum_{i=0}^n q^i}$ |
| $\overline{q^{\mathbb{Z}}}, q < 1$ | |
| $\mathbb{H}$ |
See also
$\Delta$-special functions on time scales | ||||||
 $\cos_p$ |
 $\cosh_p$ |
 $e_p$ |
 $g_k$ |
 $h_k$ |
 $\sin_p$ |
 $\sinh_p$ |
