Difference between revisions of "Delta exponential"

From timescalewiki
Jump to: navigation, search
 
(25 intermediate revisions by the same user not shown)
Line 1: Line 1:
−
Let $\mathbb{T}$ be a [[time scale]]. Define $\xi_h(z) := \dfrac{1}{h} \log(1+zh)$. Let $p \in \mathcal{R}(\mathbb{T},\mathbb{R})$ be a [[regressive_function | regressive function]]. The $\Delta$-exponential function $e_p (\cdot,\cdot;\mathbb{T}) \colon \mathbb{T} \times \mathbb{T} \rightarrow \mathbb{R}$ is defined as
+
__NOTOC__
−
 
+
Let $\mathbb{T}$ be a [[time scale]]. Let $p \in \mathcal{R}(\mathbb{T},\mathbb{C})$ be a [[regressive_function | regressive function]]. The $\Delta$-exponential function $e_p (\cdot,\cdot;\mathbb{T}) \colon \mathbb{T} \times \mathbb{T} \rightarrow \mathbb{R}$ is defined by the formula
−
$$e_p(t,s;\mathbb{T}) := \exp \left( \displaystyle\int_s^t \xi_{\mu(\tau)}(p(\tau))\Delta \tau \right)$$
+
$$e_p(t,s;\mathbb{T}) = \exp \left( \displaystyle\int_s^t \xi_{\mu(\tau)}(p(\tau))\Delta \tau \right),$$
−
 
+
where $\exp$ denotes the [http://specialfunctionswiki.org/index.php/Exponential exponential function] and $\xi_{\mu(\tau)}$ denotes the [[cylinder transformation]].  
−
It turns out that $e_p$ is the unique solution to the dynamic initial value problem
 
−
$$y^{\Delta} = py; y(s)=1.$$
 
  
 
<div align="center">
 
<div align="center">
Line 14: Line 12:
 
</div>
 
</div>
  
−
== Properties of $\Delta$-exponential Functions ==
+
=Properties=
−
For all $p,q \in \mathcal{R}$ and $t,s \in \mathbb{T}$...
+
[[Delta exponential dynamic equation]]<br />
 +
[[Semigroup property of delta exponential]]<br />
 +
[[Delta exponential with p=0]]<br />
 +
[[Delta exponential with t=s]]<br />
 +
[[Delta simple useful formula]]<br />
 +
[[Reciprocal of delta exponential]]<br />
 +
[[Product of delta exponentials with fixed t and s]]<br />
 +
[[Quotient of delta exponentials with fixed t and s]]<br />
 +
[[Relationship between delta exponential and nabla exponential]]<br />
 +
[[Relationship between delta exponential and nabla exponential]]<br />
 +
[[Relationship between nabla exponential and delta exponential]]<br />
  
 
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px">
 
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px">
−
<strong>Theorem (Semigroup property):</strong> For $p \in \mathcal{R}$ and $t,s,r \in \mathbb{T}$, the following formula holds:
+
<strong>Proposition:</strong> The following formula holds:
−
$$e_p(t,r)e_p(r,s)=e_p(t,s).$$
+
$$e_{\ominus z}(\sigma(t),s) = \dfrac{e_{\ominus z}(t,s)}{1+\mu(t)z} = -\dfrac{(\ominus z)(t)}{z} e_{\ominus z}(t,s).$$
 
<div class="mw-collapsible-content">
 
<div class="mw-collapsible-content">
−
<strong>Proof:</strong>  █
 
−
</div>
 
−
</div>
 
  
−
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px">
 
−
<strong>Theorem:</strong> Let $p \in \mathcal{R}$ and $t,s \in \mathbb{T}$, then the following formulas hold:
 
−
$$e_0(t,s)=1$$
 
−
and
 
−
$$e_p(t,t)=1.$$
 
−
<div class="mw-collapsible-content">
 
−
<strong>Proof:</strong>  █
 
 
</div>
 
</div>
 
</div>
 
</div>
  
−
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px">
 
−
<strong>Theorem:</strong> Let $p \in \mathcal{R}$ and $t,s \in \mathbb{T}$, then the following formula holds:
 
−
$$e_p(\sigma(t),s)=(1+\mu(t)p(t))e_p(t,s).$$
 
−
<div class="mw-collapsible-content">
 
−
<strong>Proof:</strong>  █
 
−
</div>
 
−
</div>
 
−
 
−
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px">
 
−
<strong>Theorem:</strong> Let $p \in \mathcal{R}$ and $t,s \in \mathbb{T}$, then the following formula holds:
 
−
$$\dfrac{1}{e_p(t,s)}=e_{\ominus p}(s,t).$$
 
−
<div class="mw-collapsible-content">
 
−
<strong>Proof:</strong>  █
 
−
</div>
 
−
</div>
 
  
−
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px">
+
=Examples=
−
<strong>Theorem:</strong> Let $p, q \in \mathcal{R}$ and $t,s \in \mathbb{T}$, then the following formula holds:
+
<center>
−
$$e_p(t,s)e_q(t,s)=e_{p \oplus q}(t,s).$$
 
−
<div class="mw-collapsible-content">
 
−
<strong>Proof:</strong>  █
 
−
</div>
 
−
</div>
 
−
 
 
−
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px">
 
−
<strong>Theorem:</strong> Let $p,q \in \mathcal{R}$ and $t,s \in \mathbb{T}$, then the following formula holds:
 
−
$$\dfrac{e_p(t,s)}{e_q(t,s)} = e_{p \ominus q}(t,s).$$
 
−
<div class="mw-collapsible-content">
 
−
<strong>Proof:</strong>  █
 
−
</div>
 
−
</div>
 
−
 
 
−
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px">
 
−
<strong>[[Relationship between delta exponential and nabla exponential|Theorem]]:</strong> Let $p \in \mathcal{R}$ and $t,s \in \mathbb{T}$, then the following formula holds:
 
−
$$\left( \dfrac{1}{e_p(\cdot,s)} \right)^{\Delta} = -\dfrac{p(t)}{e_p^{\sigma}(\cdot,s)}.$$
 
−
<div class="mw-collapsible-content">
 
−
<strong>Proof:</strong>  █
 
−
</div>
 
−
</div>
 
−
{{:Relationship between delta exponential and nabla exponential}}
 
−
{{:Relationship between nabla exponential and delta exponential}}
 
−
 
 
−
== Examples of $\Delta$-exponential Functions ==
 
−
*The [[Gaussian_bell | Gaussian bell]]
 
 
{| class="wikitable"
 
{| class="wikitable"
 
|+Time Scale $\Delta$-exponential Functions
 
|+Time Scale $\Delta$-exponential Functions
Line 134: Line 91:
 
\end{array} \right.$
 
\end{array} \right.$
 
|}
 
|}
 +
</center>
 +
 +
=See Also=
 +
[[Nabla exponential]]<br />
 +
[[Gaussian bell]]
 +
 +
=References=
 +
* {{BookReference|Dynamic Equations on Time Scales|2001|Martin Bohner|author2=Allan Peterson|prev=findme|next=Semigroup property of delta exponential}}: Definition $2.30$
 +
 +
<center>{{:Delta special functions footer}}</center>
  
−
{{:Delta special functions footer}}
+
[[Category:specialfunction]]
 +
[[Category:Definition]]

Latest revision as of 14:12, 28 January 2023

Let $\mathbb{T}$ be a time scale. Let $p \in \mathcal{R}(\mathbb{T},\mathbb{C})$ be a regressive function. The $\Delta$-exponential function $e_p (\cdot,\cdot;\mathbb{T}) \colon \mathbb{T} \times \mathbb{T} \rightarrow \mathbb{R}$ is defined by the formula $$e_p(t,s;\mathbb{T}) = \exp \left( \displaystyle\int_s^t \xi_{\mu(\tau)}(p(\tau))\Delta \tau \right),$$ where $\exp$ denotes the exponential function and $\xi_{\mu(\tau)}$ denotes the cylinder transformation.

  • Graph of $e_2(t,-1;\mathbb{Z})$.

  • Graph of $e_2(t,0;\mathbb{Z})$.

  • Graph of $e_2(t,1;\mathbb{Z})$.

Properties

Delta exponential dynamic equation
Semigroup property of delta exponential
Delta exponential with p=0
Delta exponential with t=s
Delta simple useful formula
Reciprocal of delta exponential
Product of delta exponentials with fixed t and s
Quotient of delta exponentials with fixed t and s
Relationship between delta exponential and nabla exponential
Relationship between delta exponential and nabla exponential
Relationship between nabla exponential and delta exponential

Proposition: The following formula holds: $$e_{\ominus z}(\sigma(t),s) = \dfrac{e_{\ominus z}(t,s)}{1+\mu(t)z} = -\dfrac{(\ominus z)(t)}{z} e_{\ominus z}(t,s).$$


Examples

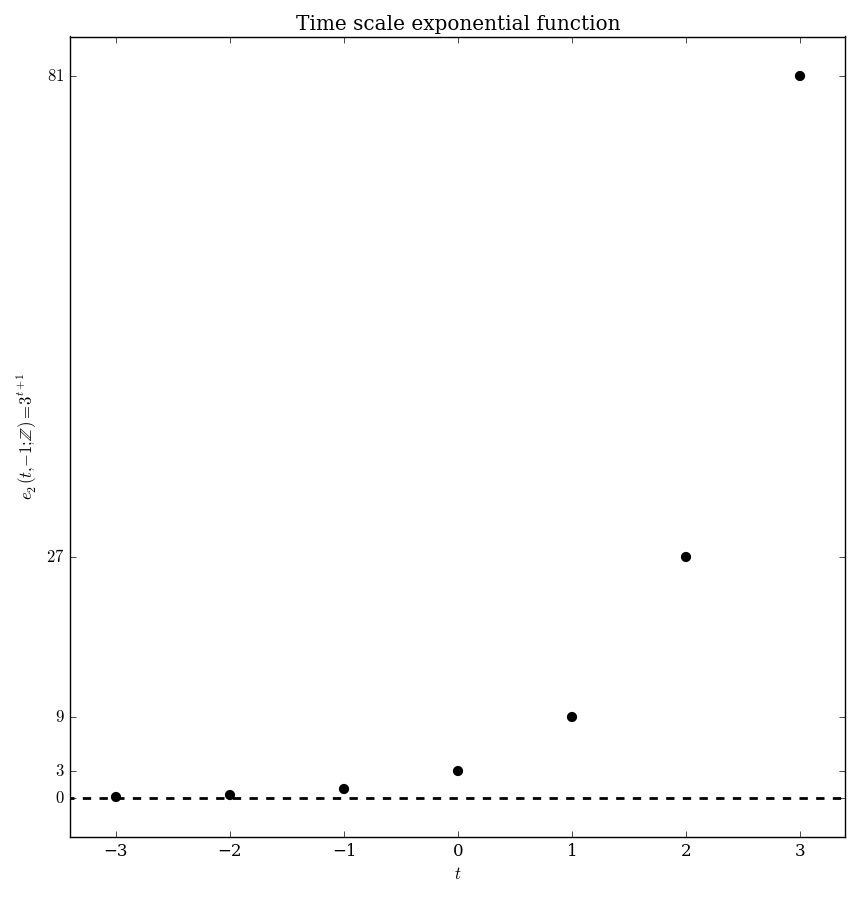
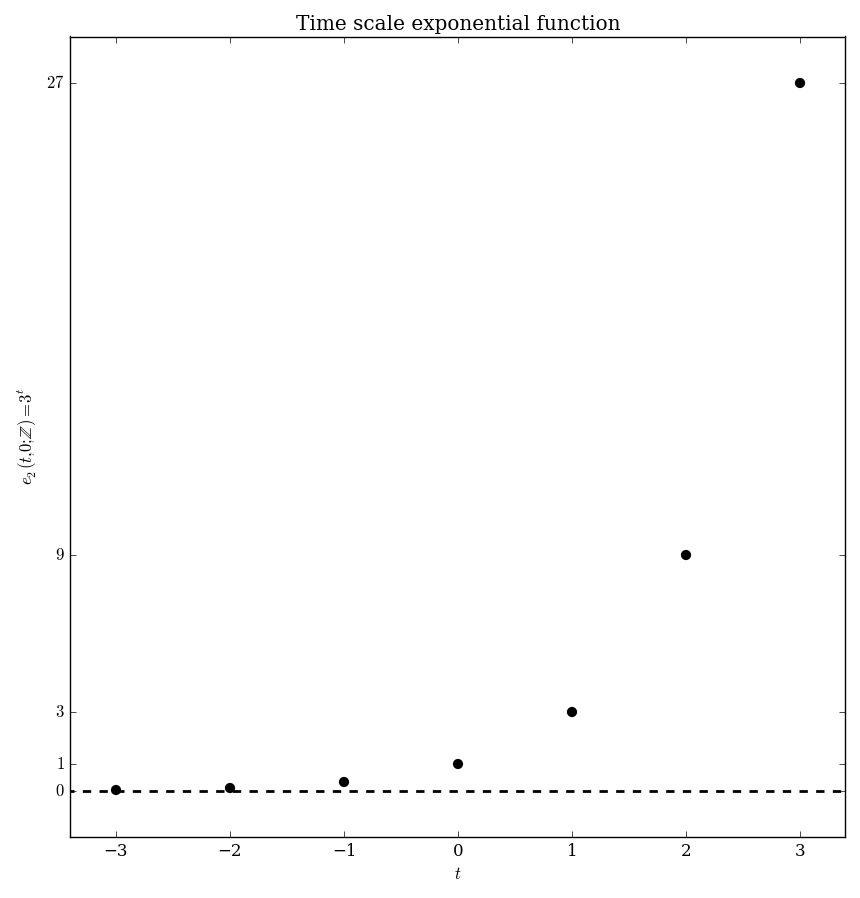
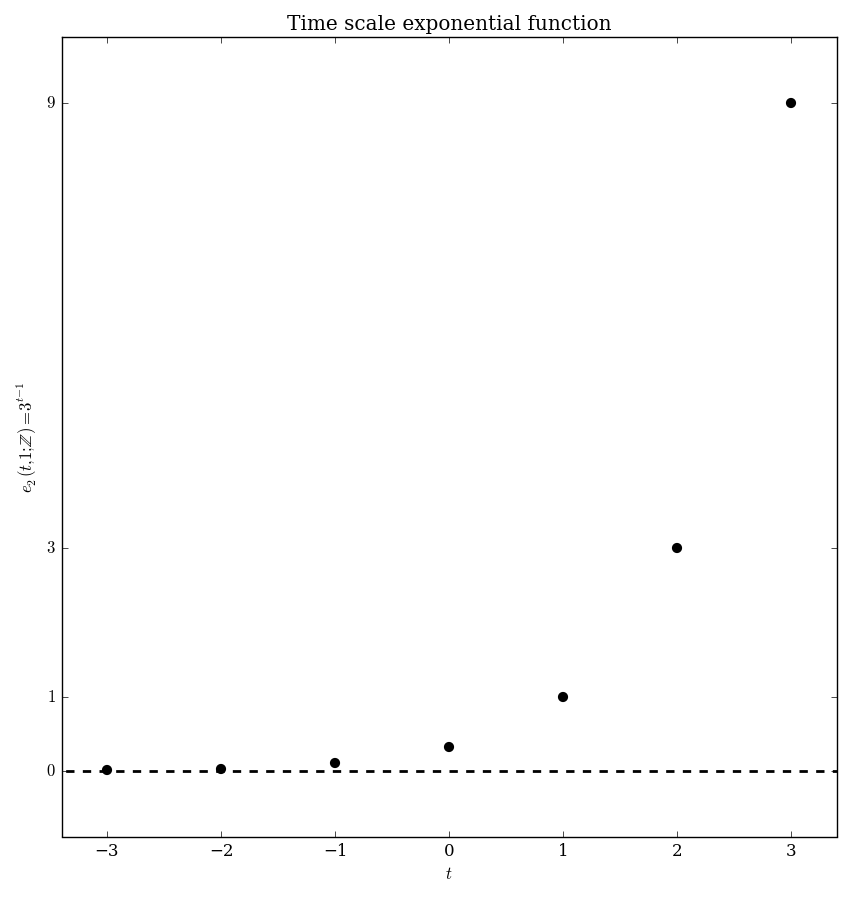
Time Scale $\Delta$-exponential Functions
$\mathbb{T}=$ $e_p(t,s)=$
$\mathbb{R}$ $e_p(t,s)= \left\{ \begin{array}{ll} \exp \left( \displaystyle\int_s^t p(\tau) d \tau \right) &; t>s \\ 1 &; t=s \\ \exp \left( -\displaystyle\int_t^s p(\tau) d\tau \right) &; t<s \end{array} \right.$
$\mathbb{Z}$ $e_p(t,s) = \left\{ \begin{array}{ll} \displaystyle\prod_{k=s}^{t-1} 1+p(k) &; t > s \\ 1 &; t=s \\ \displaystyle\prod_{k=t}^{s-1} \dfrac{1}{1+p(k)}&; t < s \end{array} \right.$
$h\mathbb{Z}$ $ e_p(t,s) = \left\{ \begin{array}{ll} \displaystyle\prod_{k=\frac{s}{h}}^{\frac{t}{h}-1} (1+hp(hk)) &; t > s \\ 1 &; t=s \\ \displaystyle\prod_{k=\frac{t}{h}}^{\frac{s}{h}-1} \dfrac{1}{1+hp(hk)} &; t < s \end{array} \right.$
$\mathbb{Z}^2$ $ e_p(t,s) = \left\{\begin{array}{ll} \displaystyle\prod_{k=\sqrt{s}}^{\sqrt{t}-1} 1 + p(k^2)(2k+1) &; t > s \\ 1 &; t=s\\ \displaystyle\prod_{k=\sqrt{t}}^{\sqrt{s}-1} \dfrac{1}{1+p(k^2)(2k+1)} &; t < s \end{array} \right.$
$\overline{q^{\mathbb{Z}}}, q > 1$ $e_p(t,s) = \left\{ \begin{array}{ll} \displaystyle\prod_{k=\log_q(s)}^{\log_q(t)-1} 1 + p(q^k)q^k(q-1) &; t > s \\ 1 &; t=s \\ \displaystyle\prod_{k=\log_q(t)}^{\log_q(s)-1} \dfrac{1}{1+p(q^k)q^k(q-1)} &; t < s \end{array} \right.$
$\overline{q^{\mathbb{Z}}}, q < 1$ $e_p(t,s) = \left\{ \begin{array}{ll} \displaystyle\prod_{k=\log_q(s)}^{\log_q(t)-1} 1 + p(q^k)q^{k-1}(1-q) &; t > s \\ 1 &; t=s \\ \displaystyle\prod_{k=\log_q(t)}^{\log_q(s)-1} \dfrac{1}{1+p(q^k)q^{k-1}(1-q)} &; t < s \end{array} \right.$
$\mathbb{H}$ $ e_p(t,s) = e_p\left( \displaystyle\sum_{k=1}^n \dfrac{1}{k}, \displaystyle\sum_{k=1}^m \dfrac{1}{k} \right) = \left\{\begin{array}{ll} \displaystyle\prod_{k=m}^{n-1} {1 + \dfrac{1}{k+1} p \left( \displaystyle\sum_{j=1}^k \dfrac{1}{j} \right)} &; t > s \\ 1 &; t=s \\ \displaystyle\prod_{k=n}^{m-1} \dfrac{1}{1 + \dfrac{1}{k+1} p \left( \displaystyle\sum_{j=1}^k \dfrac{1}{j} \right)} &; t < s \end{array} \right.$

See Also

Nabla exponential
Gaussian bell

References

$\Delta$-special functions on time scales


$\cos_p$

$\cosh_p$

$e_p$

$g_k$

$h_k$

$\sin_p$

$\sinh_p$
Retrieved from "http://timescalewiki.org/index.php?title=Delta_exponential&oldid=2314"